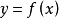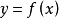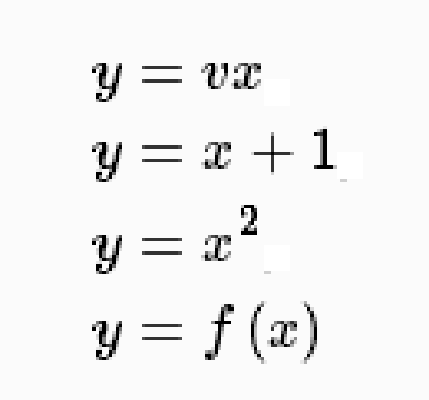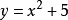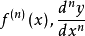顯函式

顯函式是函式的類型之一，解析式中明顯地用一個變數的代數式表示另一個變數時，稱為顯函式。

基本介紹

• 中文名：顯函式
• 外文名：explicit function
• 所屬學科：數學
• 相關概念：隱函式、顯函式求導等
• 特徵式：y=f(x)

定義

定義1

對於一個函式，如果已知自變數取某一值時，可以不必通過解方程即能求得因變數的對應值，這樣的函式叫做顯函式。或者說若y是x的函式，當直接給出y等於一個只含自變數和中間變數的解析式子時，此時y叫做自變數x的顯函式。

參考定義2

顯函式：一個函式如果能用形如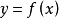的解析式表示，其中分別是函式的自變數與因變數，則此函式稱為顯函式，如等都是顯函式。

隱函式：如果由方程可確定y是x的函式，即在某個範圍記憶體在函式，使，由這種方式表示的函式是隱函式。

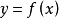
參考定義3

顯函式：自變數與因變數已經明顯分離的函式稱為“顯函式”，如等都是顯函式。

隱函式：自變數與因變數沒有明顯分離或無法分離的函式稱為“隱函式”(意思是這種函式的函式關係“隱藏”在方程之中)，如等都是隱函式，一元隱函式的一般形式是。

隱函式與顯函式的區別

如果方程f(x,y)=0能確定y與x的對應關係，那么稱這種表示方法表示的函式為隱函式。 隱函式不一定能寫為y=f(x)的形式，如x²+y²=0。因此按照函式"設x和y是兩個變數，D是實數集的某個子集，若對於D中的每個值，變數x按照一定的法則有一個確定的值y與之對應，稱變數y為變數x的(顯)函式，記作 y=f(x)"的定義，隱函式不一定是“函式”，而是“方程”。 也就是說，函式都是方程，但方程卻不一定是函式。顯函式是用y=f(x)表示的函式，左邊是一個y右邊是x的表達式 比如y=2x+1。隱函式是x和y都混在一起的，比如2x-y+1=0。有些隱函式可以表示成顯函式，叫做隱函式顯化，但也有些隱函式是不能顯化的，比如e^y+xy=1。

顯函式與隱函式的區別不是絕對的。有些隱函式可以化成顯函式，如(R為常數)可以化成；有些隱函式如雖然也確定著x,y之間的函式關係，但y不能化為x的顯函式。

顯函式求導

若可導函式的導函式仍然可導，則稱的導數為函式的二階導數，記作，或，即

或

相應地，稱為函式的一階導數。

類似地，若仍然可導，則稱的導數為函式的三階導數，記作,或。

一般地，若函式的n-1階導函式仍然可導，則稱n-1階導函式的導數為函式的n階導數，記作．或，即

或

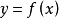函式在點處的n階導數值記作或。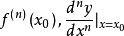

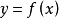
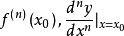
函式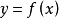的二階及二階以上的導數統稱為函式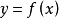的高階導數。