哈密頓圖（哈密爾頓圖）（英語：Hamiltonian graph，或Traceable graph）是一個無向圖，由天文學家哈密頓提出，由指定的起點前往指定的終點，途中經過所有其他節點且只經過一次。在圖論中是指含有哈密頓迴路的圖，閉合的哈密頓路徑稱作哈密頓迴路（Hamiltonian cycle），含有圖中所有頂點的路徑稱作哈密頓路徑（Hamiltonian path）。
基本介紹
- 中文名：哈密頓迴路
- 外文名：Hamiltonian cycle
- 說明：是一個無向圖
- 提出者：天文學家哈密頓
由來,算法,C代碼,C++代碼,
由來
天文學家哈密頓(William Rowan Hamilton) 提出，在一個有多個城市的地圖網路中，尋找一條從給定的起點到給定的終點沿 途恰好經過所有其他城市一次的路徑。
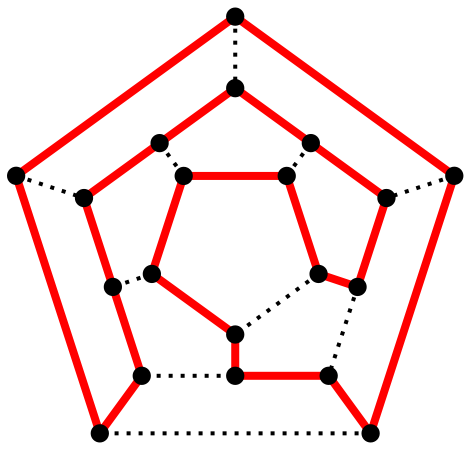哈密頓迴路
哈密頓迴路這個問題和著名的七橋問題的不同之處在於，過橋只需要確定起點，而不用確定終點。哈密頓問題尋找一條從給定的起點到給定的終點沿 途恰好經過所有其他城市一次的路徑。
算法
哈密頓路徑問題在上世紀七十年代初，終於被證明是“NP完全”的。據說具有這樣性質的問題，難於找到一個有效的算法。實際上對於某些頂點數不到100的網路，利用現有最好的算法和計算機也需要比較荒唐的時間（比如幾百年）才能確定其是否存在一條這樣的路徑。
從圖中的任意一點出發，路途中經過圖中每一個結點若且唯若一次，則成為哈密頓迴路。
要滿足兩個條件：
⒈封閉的環
⒉是一個連通圖，且圖中任意兩點可達
經過圖（有向圖或無向圖）中所有頂點一次且僅一次的通路稱為哈密頓通路。
經過圖中所有頂點一次且僅一次的迴路稱為哈密頓迴路。
具有哈密頓迴路的圖稱為哈密頓圖，具有哈密頓通路但不具有哈密頓迴路的圖稱為半哈密頓圖。
平凡圖是哈密頓圖。
⒊若以1到2、2到3、3到4、4到5、5到1，為計數規律，則各點均出現兩次；這種判斷方法在計算機編程運算中顯得尤為重要，其會精簡很多運算過程。
⒋新出爐，有待檢測的代碼如下：
%-------輸入的數據的原數據參照% v1 v2 v3 v4 v5%v1 0 20 1 11 2%v2 0 0 9 1 3%v3 0 0 0 13 8%v4 0 0 0 0 6%v5 0 0 0 0 0%以上為輸入數據的原數據參照%建議所計算的數據矩陣長度為5，不會產生bug，且不會對任何計算機造成計算負擔%輸入數據矩陣長度可以超過5，但是最初計算出的n個最小值中，重複次數超過2的點的種類只允許為一種a=[0 20 1 11 20 0 9 1 30 0 0 13 80 0 0 0 60 0 0 0 0];l=length(a)s1=infzp=infn2=1f=af(a==0)=infb=zeros(l)i1=0while i1<=l-1 [r c]=find(f==min(min(f))) b(r(1),r(1))=f(r(1),c(1)) f(r(1),c(1))=inf i1=i1+1endf1=f[rz cz]=find(b>0)pathz=[rz cz]pz=[rz;cz]p2z=zeros（2*l,1）i2z=1n2z=0while i2z<=2*l [r2z c2z]=find(pz==pz(i2z,1)) k1z=size(r2z) if k1z(1,1)>2 p2z(r2z,1)=pz(r2z,1) n2z=n2z+1 end i2z=i2z+1endif n2z==2 HHL=b zp=sum(sum(b))elsewhile min(min(f1）)~=inf if n2>2 b=snh end [r1 c1]=find(b>0) path1=[r1 c1] p1=[r1;c1] p2=zeros（2*l,1） i2=1 n2=0 while i2<=2*l [r2 c2]=find(p1==p1(i2,1) k1=size(r2) if k1(1,1)>2 p2(r2,1)=p1(r2,1) n2=n2+1 end i2=i2+1 end [r3 c3]=find(p2>0) p3=zeros(l,2) i3=0 while i3<=n2-1 if r3(1)<=l p3(r3(1),:)=path1(r(1),:) else p3(r3(1)-l,:)=path1(r3(1)-l,:) end r3(1)=[] i3=i3+1endp3(p3==0)=[]p3=reshape(p3,n2,2）p8=p2[r8 c8]=find(p8>0)p9=p8r9=r8i4=1while i4<=n2 f1(p9(r9(1),1),:)=inf f1(:,p9(r9(1),1))=inf r9(1)=[] i4=i4+1end[r4 c4]=find(f1==min(min(f1)))f1(r4,c4)=infb1=bb1(r4,c4)=a(r4,c4)i5=1p4=p3while i5<=n2b1=bb1(r4(1),c4(1))=a(r4(1),c4(1))b1(p4(1,1),p4(1,2))=0p4(1,:)=[][r5 c5]=find(b1>0)p5=[r5;c5]i6=1n6=0while i6<=2*l [r6 c6]=find(p5==p5(i6,1)) k6=size(r6） if k6(1,1)>2 n6=n6+1 end i6=i6+1endif n6>2 if sum(sum(b1))<s1 snh=[] s1=sum(sum(b1）) snh=b1 endelse if sum(sum(b1）)<zp HHL=[] zp=sum(sum(b1）) HHL=b1 endendi5=i5+1endend[rs cs]=find(HHL>0)minpaths=[rs cs]journeys=zp
註：這段代碼採用分支定界法作為編寫程式的依據，因此代碼依舊局限在算法上；而且代碼的使用對所要計算的數據是有要求的，如下：
⒈只要數據在開始計算出的n個最小值中，其重複次數超過2次的點的種類只能為一種，例如：代碼段中的數據五個最小值中其重複次數超過2次的點只有v5。
⒉數據矩陣格式要求：只允許為上三角矩陣，不支持全矩陣以及下三角矩陣的運算。
⒊代碼擴展方法請使用者獨立思考，不唯一。
⒋運算數據擴展方法，請使用者獨立思考，不唯一。
⒌此代碼為本人畢設的附加產品，不會對使用此代碼者，因理解不當或使用不當而造成的任何不良後果，付出任何責任。
⒍代碼僅供交流。
C代碼
#include<stdio.h>#include<windows.h>#include<string.h>#include<stdlib.h>#define OK 1#define ERROR 0#define TRUE 1#define FALSE 0#define MAX_VER_NUM 11//頂點的最大數#define MAX_ARC_NUM 22//邊的最大數typedef char VertexType;typedef int Status;typedef struct EdgeInfo{ VertexType v1; VertexType v2; int weight;}EdgeInfo;typedef struct ArcBox//邊所包含的信息{ int iver; struct ArcBox *ilink; int jver; struct ArcBox *jlink; int weight;//權值 int mark; char *info;}ArcBox;typedef struct VerBox//頂點所包含的信息{ VertexType data;//頂點值 ArcBox *firstedge;//指向鄰接點（邊所包含的信息）}VerBox;typedef struct Graph{ int vernum;//頂點總個數 int arcnum;//邊的總個數 VerBox vertexs[MAX_VER_NUM];//頂點信息}Graph;typedef struct StackData//棧中可存放的數據{ VertexType data; int lenght; struct StackData *pnext;}StackData;typedef struct Stack//棧用於存放已訪問過的頂點{ struct StackData *ptop; struct StackData *pbottom; }STNODE;typedef struct Stack_Arc//存方已訪問過的邊及頂點{ ArcBox *p[MAX_ARC_NUM]; int v_num[MAX_ARC_NUM];}SANode;int Visited[MAX_VER_NUM];//標記頂點是否被訪問過EdgeInfo Data[MAX_ARC_NUM]={{'A','B',324},{'A','J',419},{'A','K',328},{'A','D',241},{'A','C',556},{'A','F',703},{'A','G',521},{'B','G',391},{'B','H',230},{'B','I',356},{'B','J',220},{'C','F',642},{'C','E',337},{'D','F',829},{'D','K',334},{'E','F',581},{'E','G',1254},{'F','G',887},{'G','H',242},{'H','I',249},{'I','J',713},{'J','K',398}};//邊及權值int Count=0;//記可走邊的總數STNODE Stack;//存放已訪問過SANode Store_Arc_Ver;//存放弧的信息及頂點信息int LAV=-1,ALL=0;int Short_Len=1000000,Short_Load=0;//存放最斷最路經void CreateGraph(Graph **G);//創建圖int LocateVer(Graph G,VertexType v);//查找頂點v在圖中的位置void ShowAdjInfo(Graph *G);//查看鄰接點信息int FirstAdjVer(Graph *G,int v,ArcBox **u);//第一鄰接點int NextAdjVer(Graph *G,int v,int w,ArcBox **u);//下一鄰接點void NAV(ArcBox *p,int *n,int v,int w,ArcBox **u);//遞歸查找下一鄰接點void InitArcBox_mark(ArcBox *p);//初始化mark的值void DFSTraverse(Graph *G);//深度優先遍歷圖void DFST(Graph *G,int v);//剃歸深度優先遍歷void InitStack(void);//初始化棧void Push(VertexType c);//數據進棧void Pop(void);//出棧Status IsAdj(int *len,VertexType v);//判斷棧頂的點是否與A為鄰接點int main(){ Graph *G=NULL; CreateGraph(&G); printf("頂點的鄰接表：\n"); ShowAdjInfo(G);printf("\n\n"); printf("可走路徑結果:\n"); DFSTraverse(G);printf("\n"); printf("可走路徑總數:%d條；最短路徑為:路徑%d，長度為:%d\n\n",ALL,Short_Load,Short_Len); return 0;}void CreateGraph(Graph **G)//創建圖{ int i,j,k,w; char v1,v2; ArcBox *pnew; (*G)=(Graph *)malloc(1*sizeof(Graph)); if((*G)==NULL) { printf("動態記憶體分配失敗，程式終止！\n"); exit(-1); } (*G)->arcnum=MAX_ARC_NUM; (*G)->vernum=MAX_VER_NUM; for(i=0;i<(*G)->vernum;i++) { (*G)->vertexs[i].data='A'+i; (*G)->vertexs[i].firstedge=NULL; } for(k=0;k<(*G)->arcnum;k++) { v1=Data[k].v1; v2=Data[k].v2; w=Data[k].weight; i=LocateVer((**G),v1); j=LocateVer((**G),v2); if(i>=0&&j>=0) { pnew=(ArcBox *)malloc(1*sizeof(ArcBox)); if(pnew==NULL) { printf("動態記憶體分配失敗，程式終止！\n"); exit(-1); } pnew->iver=i; pnew->jver=j; pnew->weight=w; pnew->mark=FALSE; pnew->ilink=(*G)->vertexs[i].firstedge; pnew->jlink=(*G)->vertexs[j].firstedge; (*G)->vertexs[i].firstedge=pnew; (*G)->vertexs[j].firstedge=pnew; } else { printf("注意：*****頂點%c不存在！*****\n",i<0?v1:v2); } } return;}int LocateVer(Graph G,VertexType v)//查找頂點v在圖中的位置{ int i,result=-1; for(i=0;i<MAX_VER_NUM;i++) { if(G.vertexs[i].data==v) { result=i; break; } } return result;}void ShowAdjInfo(Graph *G)//查看鄰接點信息{ int v,w; ArcBox *u; for(v=0;v<G->vernum;v++) { printf("[%d|%c]",v,G->vertexs[v].data); for(w=FirstAdjVer(G,v,&u);w>=0;w=NextAdjVer(G,v,w,&u)) { printf("->[%d|%c|%d]",w,G->vertexs[w].data,u->weight); } InitArcBox_mark(G->vertexs[v].firstedge); printf("\n"); }}int FirstAdjVer(Graph *G,int v,ArcBox **u)//第一鄰接點{ int w=-1; ArcBox *p; p=G->vertexs[v].firstedge; (*u)=p; if(v==p->iver) { w=p->jver; p->mark=TRUE; } else if(v==p->jver) { w=p->iver; p->mark=TRUE; } return w;}int NextAdjVer(Graph *G,int v,int w,ArcBox **u)//下一鄰接點{ int n=-1; ArcBox *p; (*u)=NULL; p=G->vertexs[v].firstedge; NAV(p,&n,v,w,&(*u)); return n;}void NAV(ArcBox *p,int *n,int v,int w,ArcBox **u)//遞歸查找下一鄰接點{ if(p->mark==FALSE && (p->iver==v ||p->jver==v)) { (*u)=p; if(p->iver==v) { *n=p->jver;p->mark=TRUE; } else if(p->jver==v) { *n=p->iver;p->mark=TRUE; } else printf("下一鄰接點數據出錯，請檢查！\n"); } else { if(p->ilink!=NULL && *n==-1) { NAV(p->ilink,n,v,w,&(*u)); } if(p->jlink!=NULL && *n==-1) { NAV(p->jlink,n,v,w,&(*u)); } } return;}void InitArcBox_mark(ArcBox *p)//初始化mark的值{ p->mark=FALSE; if(p->ilink!=NULL) { InitArcBox_mark(p->ilink); } if(p->jlink!=NULL) { InitArcBox_mark(p->jlink); } return;}void DFSTraverse(Graph *G)//深度優先遍歷圖{ int v; for(v=0;v<G->vernum;v++) { Visited[v]=FALSE; InitArcBox_mark(G->vertexs[v].firstedge); } InitStack(); DFST(G,0); return;}void DFST(Graph *G,int v)//剃歸深度優先遍歷{ int w=-1,flag=1,i=0,enter=1,len=0; ArcBox *u;//鄰接點 StackData *p; Visited[v]=TRUE; Count++; Push(G->vertexs[v].data); if(Count==11&&IsAdj(&len,Stack.ptop->data)==1) { ALL++; printf("路徑%-2d：",ALL); printf("A"); p=Stack.ptop; len=len+p->lenght; if(Short_Len>len) Short_Load=ALL,Short_Len=len; while(p!=Stack.pbottom) { printf("->%c",p->data); p=p->pnext; } printf(" 總長度為：%d",len); printf("\n"); } for(w=FirstAdjVer(G,v,&u);w>=0;w=NextAdjVer(G,v,w,&u)) { enter=1; for(i=0;i<=LAV;i++) { if(Store_Arc_Ver.p[i]==u) { enter=0; break; } } if(enter==1) { Store_Arc_Ver.p[++LAV]=u; Store_Arc_Ver.v_num[LAV]=v; } if(Visited[w]==FALSE) { DFST(G,w); Visited[w]=FALSE; Count--; Pop(); } } for(LAV;Store_Arc_Ver.v_num[LAV]==v&&LAV>=0;)//還原當前頂點邊的狀態並出棧 { Store_Arc_Ver.p[LAV]->mark=FALSE; Store_Arc_Ver.p[LAV]=NULL; LAV--; }}void InitStack(void)//初始化棧{ Stack.pbottom=Stack.ptop=(StackData *)malloc(1*sizeof(StackData)); Stack.pbottom->pnext=NULL; return;}void Push(VertexType c)//數據進棧{ StackData *pnew; char v1,v2; int i; pnew=(StackData *)malloc(1*sizeof(StackData)); pnew->data=c; if(c=='A') { pnew->lenght=0; } else { v1=c; v2=Stack.ptop->data; for(i=0;i<MAX_ARC_NUM;i++) { if((v1==Data[i].v1 || v1==Data[i].v2) && (v2==Data[i].v1 || v2==Data[i].v2)) { pnew->lenght=Stack.ptop->lenght+Data[i].weight; } } } pnew->pnext=Stack.ptop; Stack.ptop=pnew; return;}void Pop(void){ StackData *p; p=Stack.ptop; Stack.ptop=p->pnext; free(p);}Status IsAdj(int *len,VertexType v)//判斷是棧頂的點是否與A為鄰接點{ int i; for(i=0;i<MAX_ARC_NUM;i++) { if((Data[i].v1==v&&Data[i].v2=='A')||(Data[i].v1=='A'&&Data[i].v2==v)) { *len=Data[i].weight; return TRUE; break; } } system("pause"); return FALSE;}/*數據的存儲結構為鄰接多重表，解題的思路是深度優遍歷再配合回溯法，代碼僅供學習交流使用。*/C++代碼
#include <queue>#include <cstdio>#include <set>#include <string>#include <stack>#include <cmath>#include <climits>#include <map>#include <cstdlib>#include <iostream>#include <vector>#include <algorithm>#include <cstring>#define max(a,b) (a>b?a:b)using namespace std;typedef long long(LL);typedef unsigned long long(ULL);const double eps(1e-8);char B[1<<15],*S=B,*T=B,ch;#define getc() (S==T&&(T=(S=B)+fread(B,1,1<<15,stdin),S==T)?0:*S++)int aa,bb; int F(){ while(ch=getc(),(ch<'0'||ch>'9')&&ch!='-'); ch=='-'?aa=bb=0:(aa=ch-'0',bb=1); while(ch=getc(),ch>='0'&&ch<='9')aa=aa*10+ch-'0'; return bb?aa:-aa;}#define N 100010int n,swp,cnt,z[N]; long long ans;#define swap(a,b) (swp=a,a=b,b=swp)#define abs(x) (x>0?x:-(x))#define max(a,b) (a>b?a:b)#define cmax(x) (ans<x?ans=x:1)struct P {int x,y,id,nx,ny;} p[N];bool operator<(const P&a,const P&b) {return a.nx<b.nx||a.nx==b.nx&&a.ny<b.ny;}class Graph{private: int et,la[N],ufs[N],tot; struct D { int x,y,v; bool operator<(const D&a)const {return v<a.v;} } d[N<<2]; struct E {int to,v,nxt;} e[N<<1]; int gf(int x) {return ufs[x]==x?x:ufs[x]=gf(ufs[x]);} void adde(int x,int y,int v) { e[++et]=(E) {y,v,la[x]},la[x]=et; e[++et]=(E) {x,v,la[y]},la[y]=et; }public: Graph() {et=1;} void add(int x,int y,int v) {d[++tot]=(D) {x,y,v};} void make() { std::sort(d+1,d+1+tot); for(int i=1; i<=n; i++)ufs[i]=i; cnt=n; for(int i=1,x,y; i<=tot; i++) if((x=gf(d[i].x))!=(y=gf(d[i].y))) { ufs[x]=y,cnt--,ans+=d[i].v, adde(d[i].x,d[i].y,d[i].v); } }} G;struct D {int x,n;} d[N];bool operator<(const D&a,const D&b) {return a.x<b.x;}#define dis(i,j) (abs(p[i].x-p[j].x)+abs(p[i].y-p[j].y))void ins(int i){ for(int t=p[i].ny; t<=cnt; t+=t&-t) if(z[t]==0||p[z[t]].x+p[z[t]].y<p[i].x+p[i].y)z[t]=i;}int query(int i){ int f=0; for(int t=p[i].ny; t>0; t-=t&-t) if(z[t]&&(f==0||p[z[t]].x+p[z[t]].y>p[f].x+p[f].y))f=z[t]; return f;}void work(){ for(int i=1; i<=n; i++)p[i].nx=p[i].x-p[i].y,p[i].ny=p[i].y; std::sort(p+1,p+1+n); for(int i=1; i<=n; i++)d[i]=(D) {p[i].ny,i}; std::sort(d+1,d+1+n); d[n+1].x=d[n].x; cnt=1; for(int i=1; i<=n; i++) { p[d[i].n].ny=cnt; if(d[i].x!=d[i+1].x)cnt++; } memset(z,0,sizeof(z)); for(int i=1,j; i<=n; ins(i++)) if(j=query(i))G.add(p[i].id,p[j].id,dis(i,j));}int main(){ n=F(); for(int i=1; i<=n; i++)p[i]=(P) {F(),F(),i}; work(); for(int i=1; i<=n; i++)swap(p[i].x,p[i].y); work(); for(int i=1; i<=n; i++)p[i].y=-p[i].y; work(); for(int i=1; i<=n; i++)swap(p[i].x,p[i].y); work(); G.make(); printf("%lld\n",ans);}/** this code is made by crazyacking* Verdict: Accepted* Submission Date: 2015-09-11-15.31* Time: 0MS* Memory: 137KB*/