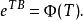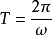弗洛凱理論是常微分方程理論的一種,討論有關周期係數線性微分方程的解答類別。
基本介紹
- 中文名:弗洛凱理論
- 外文名:Floquet theory
- 基本簡介:常微分方程理論的一種
- 套用:周期線性微分方程
- 類比:布洛赫定理
弗洛凱定理,證明,套用,
弗洛凱定理
考慮微分方程X'(t)=A(t)X(t),
其中, 矩陣
矩陣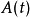 是一周期為
是一周期為 的連續周期函式。
的連續周期函式。

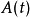

弗洛凱理論的主要定理-弗洛凱定理給出了一般線性系統的每個基本解的正規形式。它給定了一座標變換 ,其中
,其中 ,用以來轉變周期系統至有常數及實係數的傳統線性系統。
,用以來轉變周期系統至有常數及實係數的傳統線性系統。


在固態物理中,其類比的結果(推廣至三維)為布洛赫定理。
證明
由線性微分方程組的基本理論,方程 有一個基解矩陣
有一個基解矩陣 ,滿足
,滿足 ,其中
,其中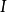 是單位矩陣。
是單位矩陣。



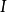
從關係式 ,容易看出,
,容易看出,


故 也是一個基解矩陣。由線性微分方程組的基本理論,必然有
也是一個基解矩陣。由線性微分方程組的基本理論,必然有 同時還能得到
同時還能得到




可知 非退化,設
非退化,設 並帶入原方程,通過一系列化簡可以得到
並帶入原方程,通過一系列化簡可以得到



這是一個常係數線性微分方程。
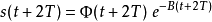


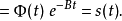
故 也是周期的。
也是周期的。