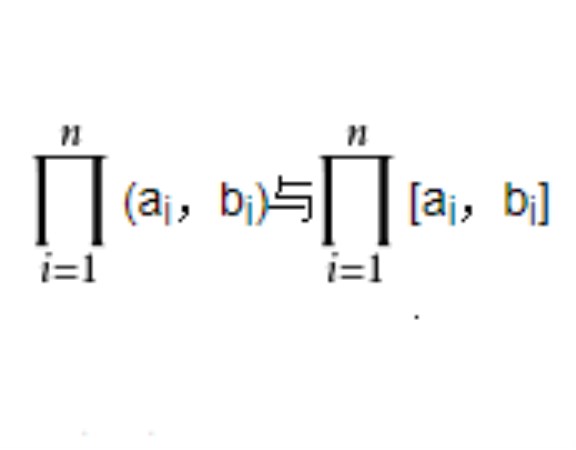n維區間是一種特殊點集,是R中區間概念的推廣。設(ai,bi),[ai,bi](i=1,2,…,n;ai,bi∈R*)是R*中的區間,積集∏i=1n(ai,bi)與∏i=1n[ai,bi],即n維空間中的集{x=(x1,x2,…,xn)|ai<xi<bi,i=1,2,…,n}與{x=(x1,x2,…,xn)|ai≤xi≤bi,i=1,2,…,n}分別稱為n維開區間與n維閉區間。
基本介紹
- 中文名:n維區間
- 所屬學科:數學
- 所屬問題:數學分析(點集論)
- 簡介:R中區間概念的推廣
基本介紹,n維區間I的容積,
基本介紹
設(ai,bi),[ai,bi](i=1,2,…,n;ai,bi∈R*)是R*中的區間,積集:


即n維空間中的集{x=(x1,x2,…,xn)|ai<xi<bi,i=1,2,…,n}與{x=(x1,x2,…,xn)|ai≤xi≤bi,i=1,2,…,n}分別稱為n維開區間與n維閉區間。
記為(a,b)與[a,b],其中a=(a1,a2,…,an),b=(b1,b2,…,bn),(ai,bi)([ai,bi])稱為(a,b)([a,b])的支區間。若所有ai,bi是實數,則稱(a,b),[a,b]為n維有界區間,否則稱為n維無界區間,上述區間統稱為n維區間。從幾何上看,二維有界區間就是矩形,三維有界區間就是長方體,因此,也把n維有界區間稱為n維長方體。特別地,當b1-a1=b2-a2=…=bn-an時,又稱為n維方體或n維方區間。例如:

就是n維開方區間,a=(a1,a2,…,an)稱為它的中心,2δ是它的棱長。n維區間(a,b)與[a,b]的n維體積是:

n維區間I的容積
n維區間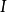 的容積都定義為:
的容積都定義為:
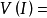
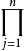
於是,當n=1時, 即為一維區間
即為一維區間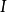 的長度,當n=2時,
的長度,當n=2時,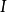 即為平面矩形面積;當n=3時,
即為平面矩形面積;當n=3時,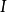 即為空間長方體的體積。
即為空間長方體的體積。

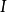
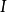
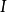
下面我們講的Rn的積分,一般都假定n≥2。
如果Rn內的點集S可以表為有限個無公共內點的n維區間 之並,即若
之並,即若



我們約定由有限個無公共內點的n維區間所成之並集,簡單地說成是n-區間有限並集。
性質1 若S是Rn的一個n-區間有限並集,則S的容積與組成S的這些區間的表示方法無關(圖1)。
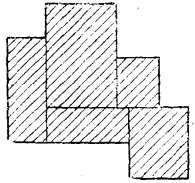 圖1
圖1證明 設S=∪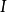 =∪J,其中
=∪J,其中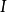 是有限個彼此無公共內點的閉區間,J也是有限個彼此無公共內點的閉區間。利用所有I與J的界面,將S分割為一系列彼此無公共內點的閉區間I'與一系列彼此無公共內點的閉區間J'。每個I'的成員與J'的每一個成員,或者無公共內點,或者內部完全重合,而所有I'的並集即為所有J'的並集。故有圖(2)
是有限個彼此無公共內點的閉區間,J也是有限個彼此無公共內點的閉區間。利用所有I與J的界面,將S分割為一系列彼此無公共內點的閉區間I'與一系列彼此無公共內點的閉區間J'。每個I'的成員與J'的每一個成員,或者無公共內點,或者內部完全重合,而所有I'的並集即為所有J'的並集。故有圖(2)
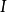
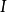
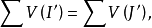
 圖2
圖2另一方面,每個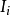 又是有限個
又是有限個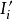 之並;每個Ji又是有限個J'i之並。 即
之並;每個Ji又是有限個J'i之並。 即
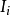
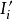

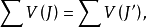
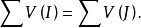
性質2 如果S與T都是n維區間的有限並集,且T S,則:
S,則:

V(S\T)=V(S) -V(T). (3)
證明因為(S\T)∪T=S,而S\T與T均為無公共內點的n維區間之並集。故由定義,有:
V(S\T)+V(T)=V(S),
此即(3)圖(3)。
 圖3
圖3性質3設S、T都是n-區間的有限並集,則有
V(S∪T)+V(S∩T)=V(S)+V(T).(4)
證明因為:SUT= [S\(S∩T)J∪[T\(S∩T)]∪[S∩T],
而S\(S∩T),T\(S∩T)和(S∩T)仍是n-區間的有限並集,它們彼此無公共內點,所以按定義,有
V(SUT)
=V[S\(S∩T)]+V[T\(S∩T)]+V(S∩T)
=V(S)-V(S∩T)+V(T)-V(S∩T)+V(S∩T)
=V(S)+V(T )-V(S∩T)
移項後,即為(4) (圖4)。
 圖4
圖4